POJ 3009 Curling 2.0 （dfs）

浏览数：25 / 时间：2015年06月09日

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 12700		Accepted: 5343

Description

On Planet MM-21, after their Olympic games this year, curling is getting popular. But the rules are somewhat different from ours. The game is played on an ice game board on which a square mesh is marked. They use only a single stone. The purpose of the game is to lead the stone from the start to the goal with the minimum number of moves.

Fig. 1 shows an example of a game board. Some squares may be occupied with blocks. There are two special squares namely the start and the goal, which are not occupied with blocks. (These two squares are distinct.) Once the stone begins to move, it will proceed until it hits a block. In order to bring the stone to the goal, you may have to stop the stone by hitting it against a block, and throw again.

技术分享
Fig. 1: Example of board (S: start, G: goal)

The movement of the stone obeys the following rules:

At the beginning, the stone stands still at the start square.
The movements of the stone are restricted to x and y directions. Diagonal moves are prohibited.
When the stone stands still, you can make it moving by throwing it. You may throw it to any direction unless it is blocked immediately(Fig. 2(a)).
Once thrown, the stone keeps moving to the same direction until one of the following occurs:
- The stone hits a block (Fig. 2(b), (c)).
  - The stone stops at the square next to the block it hit.
  - The block disappears.
- The stone gets out of the board.
  - The game ends in failure.
- The stone reaches the goal square.
  - The stone stops there and the game ends in success.
You cannot throw the stone more than 10 times in a game. If the stone does not reach the goal in 10 moves, the game ends in failure.

技术分享
Fig. 2: Stone movements

Under the rules, we would like to know whether the stone at the start can reach the goal and, if yes, the minimum number of moves required.

With the initial configuration shown in Fig. 1, 4 moves are required to bring the stone from the start to the goal. The route is shown in Fig. 3(a). Notice when the stone reaches the goal, the board configuration has changed as in Fig. 3(b).

技术分享
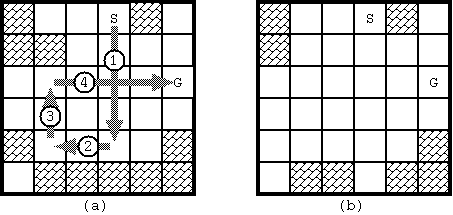
Fig. 3: The solution for Fig. D-1 and the final board configuration

Input

The input is a sequence of datasets. The end of the input is indicated by a line containing two zeros separated by a space. The number of datasets never exceeds 100.

Each dataset is formatted as follows.

the width(=w) and the height(=h) of the board
First row of the board
...
h-th row of the board

The width and the height of the board satisfy: 2 <= w <= 20, 1 <= h <= 20.

Each line consists of w decimal numbers delimited by a space. The number describes the status of the corresponding square.

0 vacant square

1 block

2 start position

3 goal position

The dataset for Fig. D-1 is as follows:

6 6
1 0 0 2 1 0
1 1 0 0 0 0
0 0 0 0 0 3
0 0 0 0 0 0
1 0 0 0 0 1
0 1 1 1 1 1

Output

For each dataset, print a line having a decimal integer indicating the minimum number of moves along a route from the start to the goal. If there are no such routes, print -1 instead. Each line should not have any character other than this number.

Sample Input

2 1
3 2
6 6
1 0 0 2 1 0
1 1 0 0 0 0
0 0 0 0 0 3
0 0 0 0 0 0
1 0 0 0 0 1
0 1 1 1 1 1
6 1
1 1 2 1 1 3
6 1
1 0 2 1 1 3
12 1
2 0 1 1 1 1 1 1 1 1 1 3
13 1
2 0 1 1 1 1 1 1 1 1 1 1 3
0 0

Sample Output

Source

Japan 2006 Domestic

题意：一种游戏，规则是：从起点S出发，在没有障碍的直线上会一直不停的行进直到遇到障碍或到达终点G停止。在遇到障碍时，要停在障碍前，然后障碍就会消除，然后重新选择四个方向行进。输入图中2代表起点，3代表终点，0代表无障碍区域，1代表障碍区域。求要从起点到达终点最少需要停多少次。

解析：dfs。

PS：C++交的结果是RE，G++是AC

AC代码：

#include<iostream>
#include<stdio.h>
#include<stdio.h>
#include<string.h>
using namespace std;
int vis[25][25];
int n,m;
int sx,sy,ex,ey;
int flag,minstep;        //flag标记是否找到路径,minstep记录总的步数
int d[4][2] = {1,0,0,1,-1,0,0,-1};   //移动的方向

void dfs(int x, int y, int step){    //当前位置，步数
    int dx, dy;
    if(step >= 10) return ;
    for(int i=0; i<4; i++){
        if(!vis[ x+d[i][0] ][ y+d[i][1] ]){
            dx = x;
            dy = y;
            while(!vis[ dx+d[i][0] ][ dy+d[i][1] ]){    //判断下一个节点是否是障碍
                dx = dx + d[i][0];                      //符合条件才加
                dy = dy + d[i][1];
                if(dx == ex && dy == ey){
                    if(minstep > step + 1) minstep = step + 1;
                    flag = 1;
                    return ;
                }
                if(dx < 0 || dx >= n || dy < 0 || dy >= m) break;  //判断越界
            }
            if(dx >= 0 && dx < n && dy >= 0 && dy < m && step + 1 < 10){    //与障碍撞上后
                vis[ dx+d[i][0] ][ dy+d[i][1] ] = 0;      //去除障碍
                dfs(dx, dy, step+1);
                vis[ dx+d[i][0] ][ dy+d[i][1] ] = 1;      //回溯
            }  
        }
    }
}

int main()
{
    #ifdef sxk
        freopen("in.txt", "r", stdin);
    #endif // sxk


    int i,j;
    while(scanf("%d%d", &m, &n)!=EOF && !(!m && !n)){
        memset(vis, 0, sizeof(vis));              //不加这句，竟然WA
        for(i=0; i<n; i++)
            for(j=0; j<m; j++){
                scanf("%d", &vis[i][j]);
                if(vis[i][j]==2){
                    sx = i;
                    sy = j;
                    vis[i][j] = 0;
                }
                else if(vis[i][j]==3){
                    ex = i;
                    ey = j;
                    vis[i][j] = 0;
                }
            }
        minstep = 99999999;
        flag = 0;
        dfs(sx, sy, 0);
        if(!flag) minstep = -1;
        printf("%d\n", minstep);
    }
    return 0;
}

郑重声明：本站内容如果来自互联网及其他传播媒体，其版权均属原媒体及文章作者所有。转载目的在于传递更多信息及用于网络分享，并不代表本站赞同其观点和对其真实性负责，也不构成任何其他建议。

POJ 3009 Curling 2.0 （dfs）